µMAG Standard Problem #4 results

See the problem specification.

Disclaimer

µMAG could not succeed without the contributions of colleagues working outside NIST. Although we value the contributions made by these colleagues, NIST does not necessarily endorse the views expressed or the data presented in the submitted solutions shown below.

Solution directory

Submitted Solution: Massimiliano d’Aquino, Claudio Serpico, and Giovanni Miano

Date:: November 20, 2005.
From:: Massimiliano d’Aquino, Claudio Serpico, and Giovanni Miano
Dipartimento di Ingegneria Elettrica, Università degli Studi di Napoli “Federico II”, I-80125 Napoli – Italy
Contact:: Massimiliano d’Aquino

A 3D micromagnetic code has been used for these calculations. The Landau-Lifshitz-Gilbert (LLG) equation has been solved with a semi-discretized approach.

The spatial discretization has been performed with the finite difference method. The magnetic body has been subdivided into a collection of N rectangular prisms with edges parallel to the coordinate axes (N = N_x × N_y × N_z, where N_x, N_y, N_z are the number of cells along the axes x, y, z respectively). The magnetization has been assumed uniform within each cell.

The exchange field has been computed by means of a 7-point laplacian discretization, which is second order accurate in space. The Neumann boundary condition has been taken into account on the boundary cells. The magnetostatic field has been written as a discrete convolution whose kernel was obtained by means of generalization to prism (non cubic) cells of the formulas proposed in Ref. [1] for cubic cells. Such discrete convolution has been computed by using 3D Fast Fourier Transform with zero-padding algorithm.

The time integration of the (spatially) semi-discretized LLG equation has been performed by using the (implicit) mid-point rule numerical technique. This technique is unconditionally stable and second order accurate with respect to the time step. In addition, when the mid-point rule scheme is applied to the LLG equation, it preserves the fundamental properties of the LLG dynamics, regardless of the time-step:

Magnetization magnitude conservation at each spatial location.
LLG Lyapunov structure (for constant in time external field), namely the magnetic free energy is a decreasing function of time.

Moreover, in the case of zero damping α=0, the free energy is exactly preserved regardless of the time step and the hamiltonian structure of the LLG equation is preserved up to third-order with respect to the time step. Details about the application of the mid-point rule to micromagnetic simulations can be found in Ref. [2].

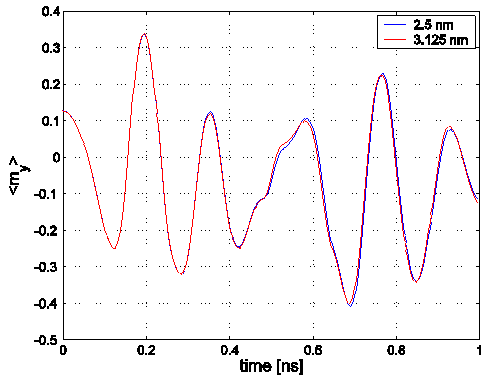
In all the computations, the time-step was constant and set to 2.5 ps. The magnetization has been assumed to have reached an equilibrium state when the maximum value of the normalized torque was less than 10^-5.

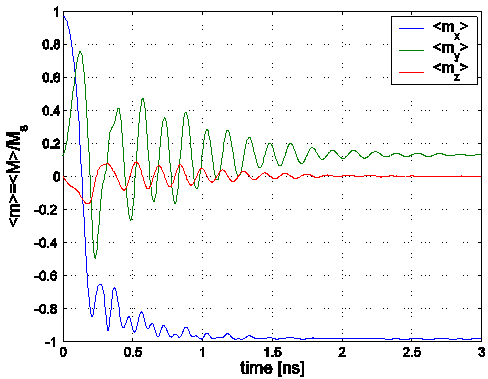
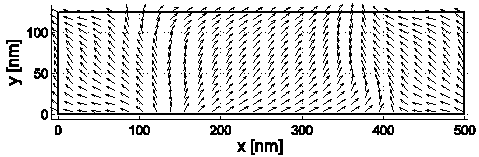
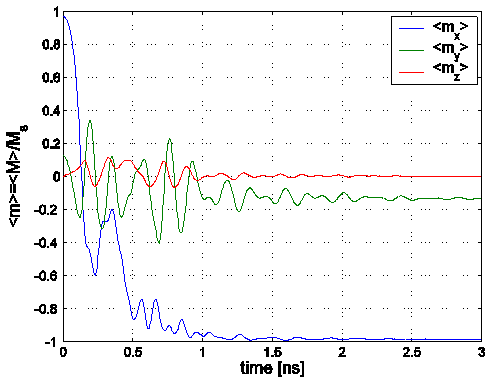
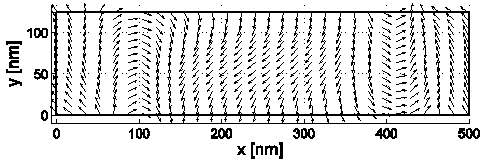
Results:

Field applied at 170° from the x-axis (Field 1):
The cell size has been chosen as 3.125 nm × 3.125 nm × 3 nm. Therefore, the thin-film has been subdivided into N = N_x × N_y × N_z = 160 × 40 × 1 = 6400 prism cells. Next we report a plot of the spatially averaged magnetization vs. time

and an image of the magnetization when < m_x > first crosses zero.
Field applied at 190° from the x-axis (Field 2):
The cell size has been initially chosen as 3.125 nm × 3.125 nm × 3 nm. Therefore, the thin-film has been subdivided into N = N_x × N_y × N_z = 160 × 40 × 1 = 6400 prism cells. Analogously as before, we report a plot of the spatially averaged magnetization vs. time

and an image of the magnetization when < m_x > first crosses zero.

To investigate the dependence of the numerical solution on the mesh size, the same problem has been simulated with a smaller cell size, namely 2.5 nm × 2.5 nm × 3 nm. Therefore, the thin-film has been subdivided into N = N_x × N_y × N_z = 200 × 50 × 1 = 10000 prism cells. Next, we report the plot of < m_y > vs. time with cell size 3.125 nm and 2.5 nm.

Data:

Field 1: Time series for 2.5 nm mesh, Time series for 3.125 nm mesh, and Vector data for 3.125 nm mesh at < m_x > = 0.
Field 2: Time series for 2.5 nm mesh, Time series for 3.125 nm mesh, and Vector data for 3.125 nm mesh at < m_x > = 0.

Time series data contain 4 columns: time (ns), m_x, m_y, m_z. Vector data has 6 columns: x, y, z coordinates (in meters), and m_x, m_y, m_z vector components.

References:

[1] M.E. Schabes and A. Aharoni, Magnetostatic Interaction Fields for a Three-Dimensional Array of Ferromagnetic Cubes, IEEE Transactions on Magnetics 23, n. 6 (1987), 3882-3888.
[2] M. d’Aquino, C. Serpico, G. Miano, Geometrical integration of Landau-Lifshitz-Gilbert equation based on the mid-point rule, Journal of Computational Physics 209 (2005), 730-753.

Please send comments to donald.porter@nist.gov and join the µMAG discussion e-mail list.

Site Directory

µMAG organization / NIST CTCMS / donald.porter@nist.gov

11-NOV-2021