


Next: Appendix: Liquid Solder Hydrostatic
Up: MECHANISM OF MOTION OF
Previous: ACKNOWLEDGMENTS
At any given point on the fiber-liquid solder-vapor triple line, the local
capillary force 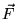 has magnitude equal to the surface tension
has magnitude equal to the surface tension
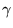 ,
and direction perpendicular to the triple line tangent vector
,
and direction perpendicular to the triple line tangent vector
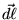 .
The total force on the fiber is equal to the integral of this quantity
around both loops of the triple line TL, so that
.
The total force on the fiber is equal to the integral of this quantity
around both loops of the triple line TL, so that
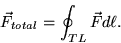 |
(2) |
The local capillary force vector 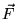 satisfies the following
constraints:
satisfies the following
constraints:
- 1.
- It is at an angle
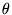 (the contact angle) to the fiber tangent
surface, so
(the contact angle) to the fiber tangent
surface, so
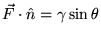 where
where 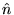 is the
outward fiber surface normal of unit length.
is the
outward fiber surface normal of unit length.
- 2.
- It is perpendicular to edge tangent vector
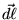 ,
so
,
so
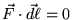 .
.
- 3.
- Its magnitude
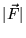 is
is 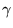 ,
the surface tension.
,
the surface tension.
- 4.
- With the edge integral making a clockwise loop
around
the fiber, the solder droplet is always on the left, so for
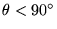 ,
,
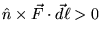 (<0 for
(<0 for
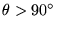 ).
).
In cylindrical coordinates transformed so the fiber centerline is the
z-axis,
its surface will be defined by r=R, so 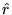 can be used as the
surface
normal
can be used as the
surface
normal 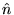 .
Based on this, we can rewrite the first three above
conditions for
.
Based on this, we can rewrite the first three above
conditions for 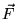 as three simultaneous equations for the three
components of
as three simultaneous equations for the three
components of 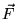 as follows:
as follows:
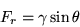 |
(3) |
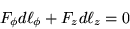 |
(4) |
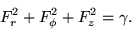 |
(5) |
(Note that
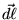 has no r-component.) We then substitute 3 into
5, and 4 into 5, and solve for
has no r-component.) We then substitute 3 into
5, and 4 into 5, and solve for 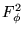 and Fz2 to give
and Fz2 to give
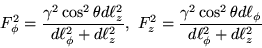 |
(6) |
which simplify, satisfying condition 4, to
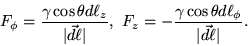 |
(7) |
The components of 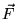 given by equations 3 and 7 are
integrated using equation 2 to give the capillary force on the fiber.
given by equations 3 and 7 are
integrated using equation 2 to give the capillary force on the fiber.



Next: Appendix: Liquid Solder Hydrostatic
Up: MECHANISM OF MOTION OF
Previous: ACKNOWLEDGMENTS
Adam Clayton Powell IV
1998-06-08
![]() satisfies the following
constraints:
satisfies the following
constraints:
![]() can be used as the
surface
normal
can be used as the
surface
normal ![]() .
Based on this, we can rewrite the first three above
conditions for
.
Based on this, we can rewrite the first three above
conditions for ![]() as three simultaneous equations for the three
components of
as three simultaneous equations for the three
components of ![]() as follows:
as follows: