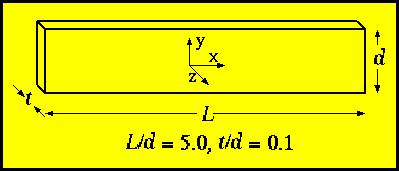µMAG Standard Problem #2

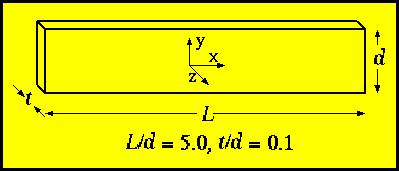
Problem suggested by Tom Koehler, IBM Almaden, ret., and proposed by H. Neal Bertram, Alfred Liu, and Chris Seberino, Center for Magnetic Recording Research, University of California at San Diego.

Please send comments to rmcmichael@nist.gov and join the µMAG discussion e-mail list for ongoing discussion.

A set of solutions have been submitted.

Specifications

This standard micromagnetic problem includes both magnetostatic and exchange energies, but has the advantage of only one scaled parameter. If crystalline anisotropy is neglected and the geometry is fixed, scaling of the static micromagnetic equations (Brown's equations) yield a hysteresis loop which depends only on the scaled geometry to the exchange length when expressed as M/M_s versus H/H_m, where H_m = M_s (SI) or 4piM_s (cgs emu). The exchange length is l_ex = (A/K_m)^1/2, where A is the exchange stiffness constant and K_m is a magnetostatic energy density, K_m = ¹/₂µ₀M_s² (SI) or 2piM_s² (cgs emu).

The field should be applied in the [1,1,1] direction, approximately 54.74 degrees ( arccos[1/root(3)] ) from each of the coordinate axes. This field orientation is chosen to avoid potential symmetry breaking problems.

Geometry:

Let us take a thin film of thickness t, width d, and length L. We suggest to make the problem virtually 2D by choosing t/d = 0.1, and to obtain interesting non-uniform reversal modes, L/d = 5.

Material parameters:

The magnetostatic exchange length, l_ex
Zero magnetocrystalline anisotropy

Desired output for comparison:

Calculated as a function of d/l_ex, with aspect ratios held constant at t/d = 0.1 and L/d = 5.0,

Coercivity (H_c/H_m, the magnitude of the field at which the projection of the magnetization along the field, M_x+ M_y+ M_z, is zero.)
Remanence ( M_x/M_s, M_y/M_s, M_z/M_s, at H = 0)

Please see the µMAG standard problem strategy page for information on publicizing your results.

Comments:

If we all begin with d/l_ex = 0.1 then the film will switch in the plane uniformly with the Stoner-Wohlfarth result for the coercivity. As we increase d/l_ex, say to d/l_ex = 2, simple nonuniform rotation will occur. For d/l_ex > 2 complex vortex formation will begin to occur and both the remanence and coercivity will become very small. Also, eventually non uniform magnetization over the film thickness will occur. We all should agree when d/l_ex = 0.1, but it will be interesting to see as we increase d/l_ex when our results diverge.

A ``2¹/₂-D'' approach, where the particle is discretized by a 2-D mesh or grid with 3-D spins, is expected to be sufficient as long as t is less than l_ex. For larger particles, a three dimensional discretization may be necessary.

The results should be shown to be independent of the discretization size.

Site Directory

µMAG organization / NIST CTCMS / rmcmichael@nist.gov
09-FEB-1998