µMAG Standard Problem #4 results

Disclaimer

Solution directory

• Comparisons
• G. Albuquerque, J. Miltat and A. Thiaville
• R. D. McMichael, M. J. Donahue, D. G. Porter, and J. Eicke
• Liliana Buda, Lucian Prejbeanu, Ursula Ebels and Kamel Ounadjela
• E. Martinez, L. Torres and L. Lopez-Diaz
• José L. Martins and Tania Rocha
• P.E. Roy and P. Svedlindh
• Massimiliano d’Aquino, Claudio Serpico, and Giovanni Miano
• Dmitri Berkov
• M. J. Donahue and D. G. Porter
• Rasmus Bjørk, E. B. Poulsen and A. R. Insinga

Submitted Solution: R. D. McMichael, M. J. Donahue, and D. G. Porter

Date:

September 27, 2000.

From:

R. D. McMichael, M. J. Donahue, and D. G. Porter.
National Institute of Standards and Technology, Gaithersburg, MD 20899
J. Eicke
Institute for Magnetics Research,
George Washington University, Washington, DC 20052

Contact:

Bob McMichael

We used the OOMMF public code to compute solutions to standard problem #4. The mesh was a 2D square grid with 3D spins interacting through the exchange interaction and magnetostatic fields. Magnetostatic energy was computed under the assumption that the magnetization was uniform within each cell, and the field due to the magnetostatic charges at the cell boundaries was averaged over each cell. Except where noted below, the exchange energy was calculated using a "eight-neighbor dot product" representation. The maximum time step was set at 0.2 ps, but smaller time steps are taken when necessary to keep the error within certain bounds. See the OOMMF documentation for mmsolve for details on the solver.

Results:

• For the first part of the problem, with the field applied 170° from the x-axis (Field 1), we used 2.5 nm cell size and maximum time steps of 0.2 ps. The maximum angle between neighboring spins on the lattice was 22.3° at 0.298 ns.

  
  a plot of the spatially averaged magnetization

  
  and an image of the magnetization when M_x first crosses zero.

• For the second part of the problem, with the field applied 190° from the x-axis (Field 2), we initially used 2.5 nm cell size and a maximum time step of 0.2 ps. The maximum angle between neighboring spins on the lattice was 71.9° at 0.571 ns. For comparison, using slightly larger 3.125 nm cells, the maximum angle was 118°. Finally, the results shown below are from a calculation done with 3.125 nm cells using a "four-angle" exchange energy representation. The four-angle representation is based on the assumption that the magnetization rotates uniformly between spins. Under this assumption, the exchange energy is proportional to the square of the angle between neighboring spins. Unlike the dot product representation, the four-angle has the property of providing an increasingly stronger aligning torque as the angle between spins increases up to 180°. Using the four-angle representation, the maximum angle between spins was 48.1°. [M. J. Donahue and R. D. McMichael, Physica B 233, 272 (1997).]

  
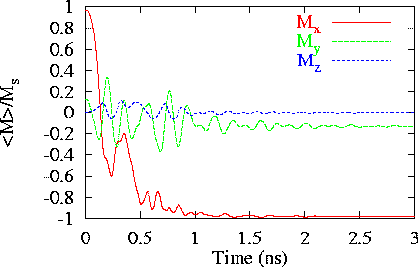
  A plot of the spatially averaged magnetization calculated with 3.125 nm cells and the four-angle exchange representation.

  
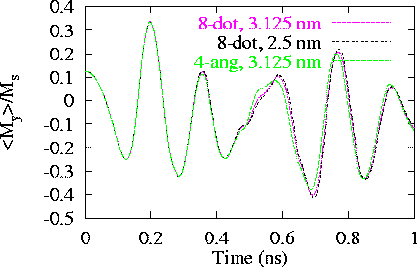
  Plots of M_y calculated with 3.125 nm and 2.5 nm grids using the eight-neighbor dot product exchange representation and with a 3.125 nm grid using the four-angle exchange representation.

  
  and an image of the magnetization when M_x first crosses zero.

Raw data:

Please note that the following data follows a coordinate system that is rotated 90° from the specified coordinate system. Vector data is in the ovf format.

• Field 1: Time series and Vector data at M_x=0.
• Field 2: Time series, 3.125 nm cells, 4-angle exchange and Vector data at M_x=0.