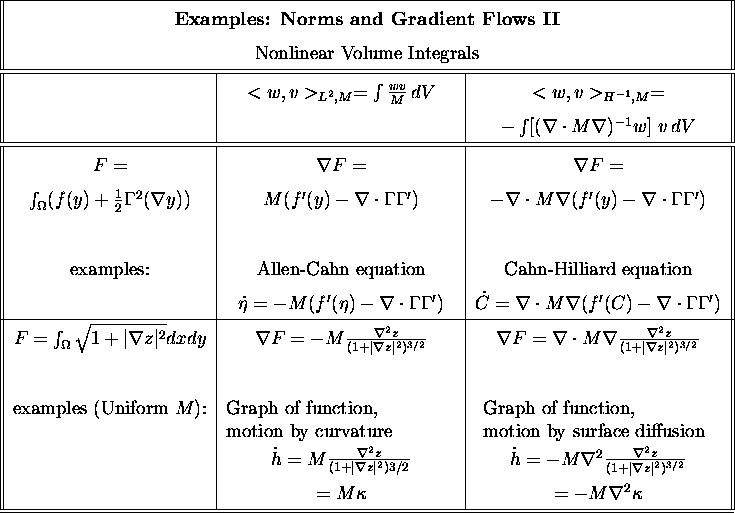
A common form of the free energy density is
,
where 
is the free energy density for a homogeneous (gradient
everywhere zero)
system.
The field 
has not been specified, but presently will be taken as a
globally
conserved quantity such as concentration in a closed system; or as a
non-conserved quantity
like order.
For conserved quantities, is the function on which a common tangent
construction is
made, and it may have several minima and need not have continuous derivatives
everywhere.
The dependence of the function 
on the direction of the
gradient 
is directly proportional to that of the orientation
dependence of the anisotropic interfacial energy
density[1].
For this case, equation 7 becomes:
For isotropic interfaces, 
:
