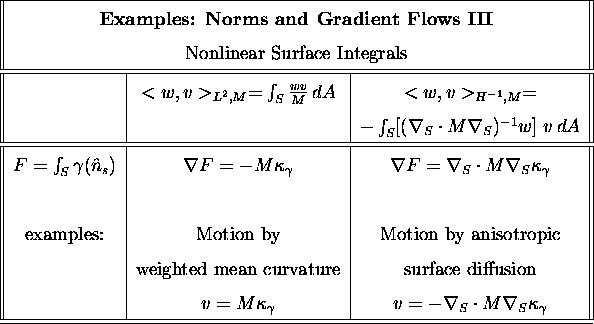
Interfacial motion arises in two different ways: 1) as the motion of two-dimensional level sets during gradient flow of an integral over volume; 2) as a velocity normal to a surface, where the velocity is gradient flow of a surface integral. The first method is discussed in Section 3.2.1.
The second approach has been discussed above and appears in tables I and in table II
in small slope approximation and as the graph of a function z(x,y), respectively, for
isotropic surfaces.
For anisotropic surfaces, the energy functional is a surface integral:
.
The interfacial surface energy density, 
, does not necessarily
have continuous derivatives of the surface orientation 
; in fact,
need not be continuous.
Furthermore, the surface S can have edges and corners where its derivatives are discontinuous;
furthermore it can have flat facets.
In this case, the variational derivative is the instantaneous increase in interfacial area due
to a normal velocity v, weighted by the surface energy density, and is called the `weighted
mean curvature' 
[6].
When 
is isotropic, is equal to 
,
where 
is the geometric mean curvature.
In two dimensions, 
, when is
twice differentiable with respect to the polar angle 
, which is the the Herring
formulation[7].
Generally,
,
where 
is the Cahn-Hoffman capillarity
vector[6, 8, 9, 4].
The anisotropic version of gradient flow for the surface integrals is summarized
in the following table:
