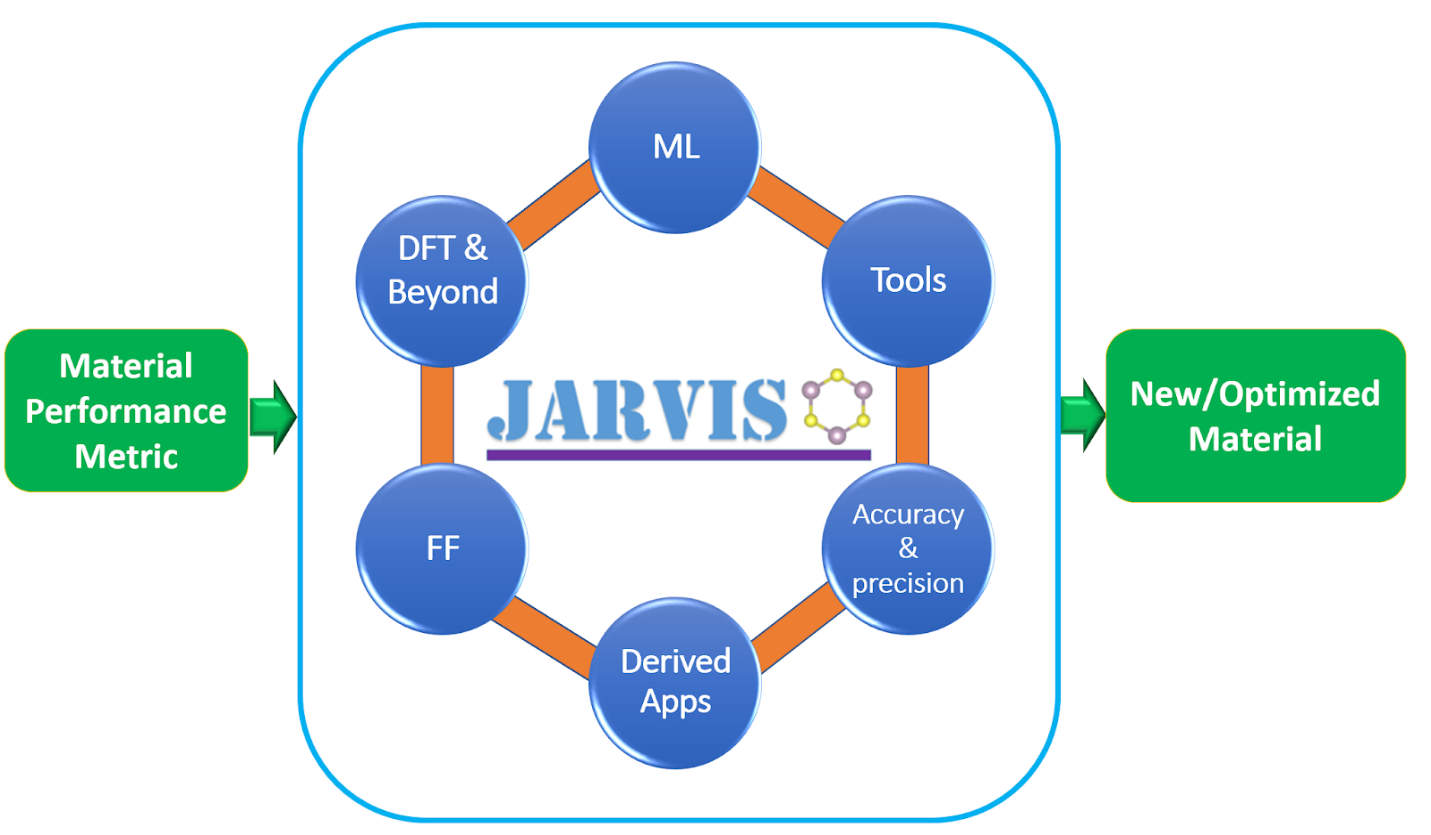
Introduction
NIST-JARVIS homepage https://jarvis.nist.gov/.
This project is a part of Materials-genome initiative at NIST.
JARVIS (Joint Automated Repository for Various Integrated Simulations) is a repository designed to automate materials discovery and optimization using:
- Classical force-field,
- Density functional theory,
- Machine learning calculations and
- Experiments.
Find more details https://www.nature.com/articles/s41524-020-00440-1 .